I’ve written two textbooks,
-
An Invitation to Analytic Combinatorics: From One to Several Variables
S. Melczer.
Springer Texts & Monographs in Symbolic Computation, 2021. -
Analytic Combinatorics in Several Variables, 2nd Edition
R. Pemantle, M. C. Wilson, and S. Melczer.
Cambridge Studies in Advanced Mathematics, 2024.
The first textbook is an introductory treatment to analytic combinatorics in several variables, suitable for graduate students and those new to the area, while the second is a more advanced treatment making extensive use of homology, multivariate residues, and similar constructions.
See also my notes on enumeration.ca for an elementary introduction to enumerative combinatorics.
News / Events
Analytic Combinatorics in Several Variables, 2nd Edition OUT NOW
Published February 2024
FPSAC 2025 (Plenary Speaker), July 2025
At Hokkaido University, Japan
AofA 2025 (Organizing Committee Co-Chair), May 2025
At the Fields Institute, Toronto
AofA 2024 (Program Committee), June 2024
At the University of Bath, UK
An Invitation to Analytic Combinatorics hits 10k chapter downloads
On SpringerLink digital service (not including hard copies sold)
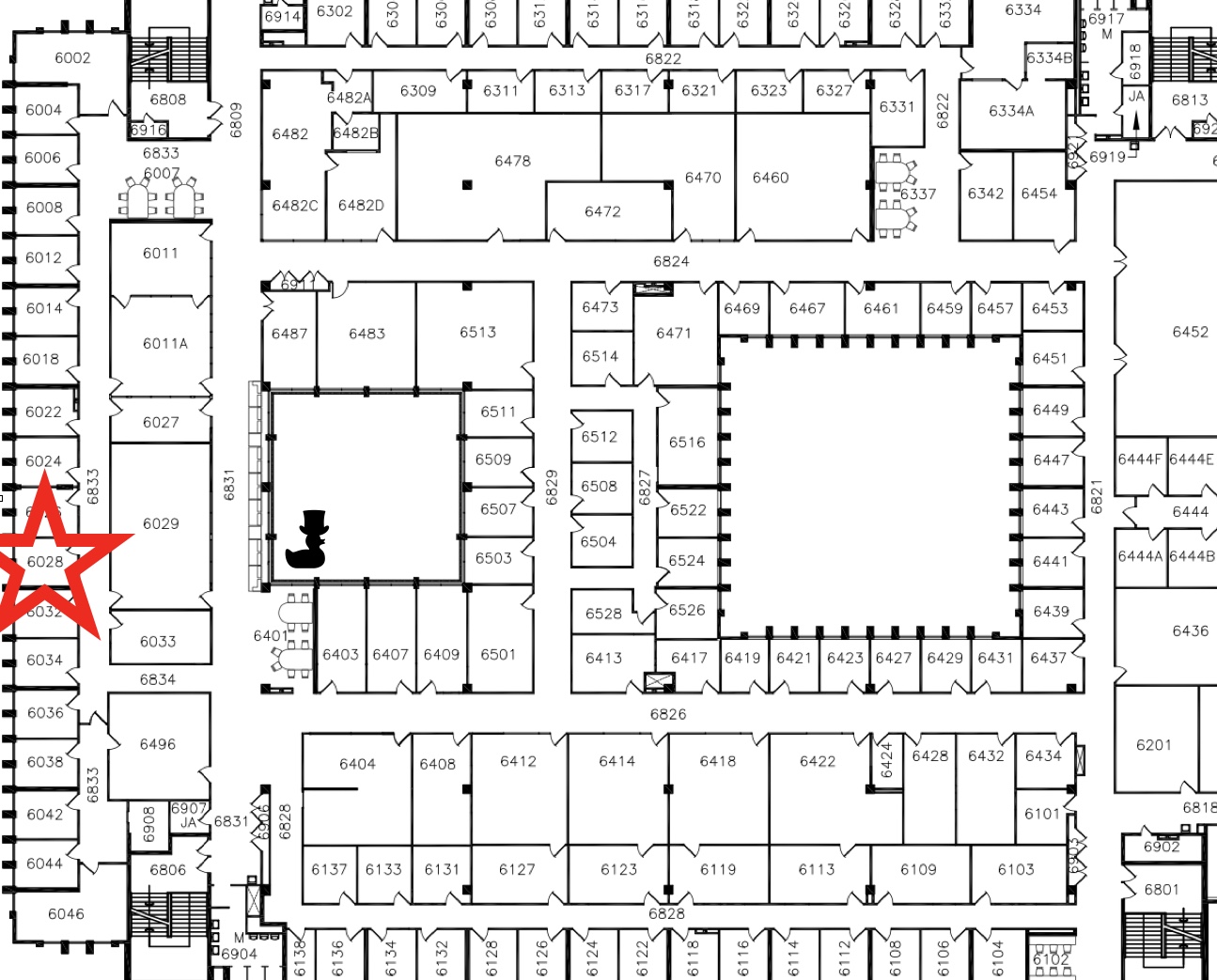
Map to Office
My office is MC 6028 (click for a larger version).
Coauthors
Yuliy Baryshnikov, Alin Bostan, Mireille Bousquet-Mélou, Sophie Burrill, Jeremy Chizewer, Julien Courtiel, Ruiwen Dong, Éric Fusy, Torin Greenwood, Benjamin Hackl, Kevin Hyun, Veronika Irvine, Manuel Kauers, Jessica Khera, Alexander Kroitor, Kisun Lee, Andreas Lenz, Erik Lundberg, Andrew Luo, Marc Mezzarobba, Marcus Michelen, Marni Mishna, Somabha Mukherjee, J. Ian Munro, Greta Panova, Robin Pemantle, Ava Pun, Kilian Raschel, Cyrus Rashtchian, Frank Ruskey, Tiadora Ruza, Bruno Salvy, Éric Schost, Jesse Selover, Paul H. Siegel, Josip Smolčić, Catherine St-Pierre, Armin Straub, Mark Wilson, Elaine Wong